Des-blog

In this week’s prompt we asked the fellows give this activity a makeover, increasing its depth, demand, or delight, by adding just one screen.

Though the makeover screens varied widely depending on lesson objective and course level, there were commonalities in how the fellows used Desmos to add value.

• They connected representations.
  
• They gave students opportunities to be right and wrong in different, interesting ways.
  
• They created activities that are easy to start and difficult to finish.
  
• They created objects that promote mathematical conversations between teachers and students.
  

(These are all elements in our activity building code.)

Connect representations.

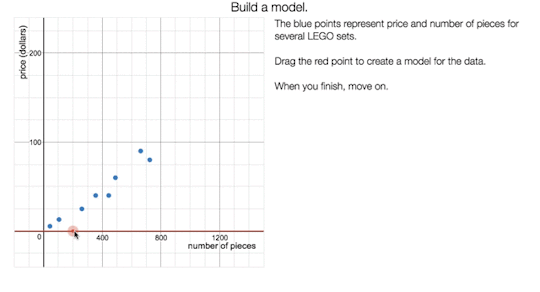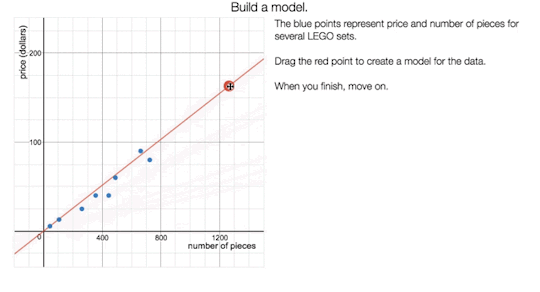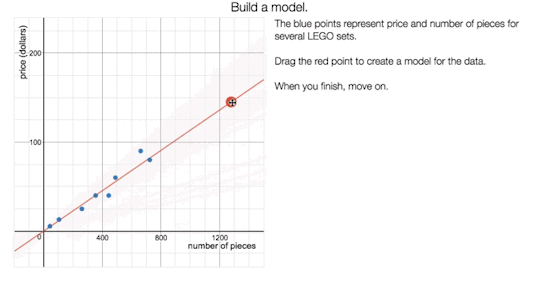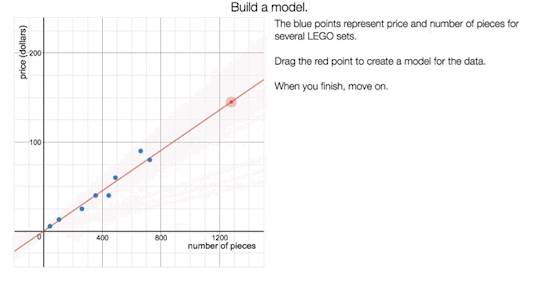
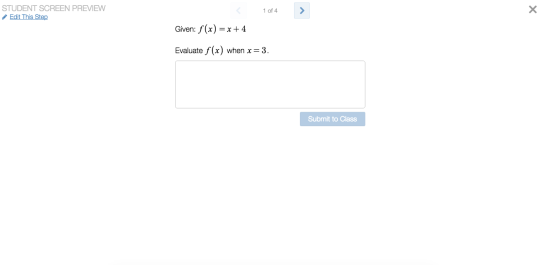
Dan Anderson, Stephanie Blair, Sarah Blick Vandivort, Meg Craig, Nolan Doyle, Adam Poetzel, Julie Reulbach, Linda Saeta, and Suzanne von Oy all used a graph to help students connect the algebraic representation of functions to the graphical representations of functions. Directions on these screens asked students to represent various values of a given function. The way in which students would respond ranged from less formal representations such as dragging or sketching points to the more formal representation of plotting a point by typing its coordinates. Several of the fellows also made use of color and styling to help students interpret a graph or display understanding.

Give students opportunities to be right and wrong in different, interesting ways.

Dave Sabol and Jenn Vadnais included a table of values to help students reason about function operations and composition. In Jade White’s screen students can take a numerical or algebraic approach allowing them to get to the answer in a number of ways, creating an opportunity for rich class discussions.

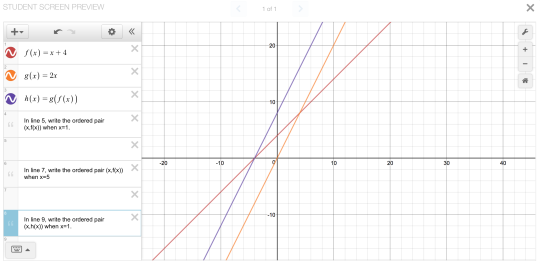
Create activities that are easy to start and difficult to finish.

Mark Alvaro and Dave Sabol added challenge screens to our original activity, making it easy to start and difficult to finish.

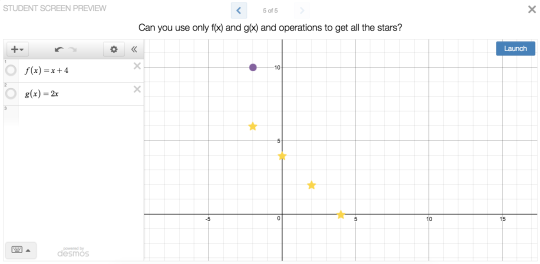
Try your hand at Dave’s Marbleslides challenge here.

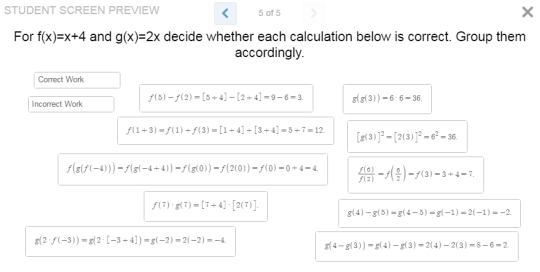
Create objects that promote mathematical conversations between teachers and students.

Serge Ballif, Nick Corley, Dan Henrikson, Paul Jorgens, Scott Miller, and Anna Scholl created screens that promote mathematical conversations between teachers and students by including problems designed to expose and confront naive conceptions that students may have. An example of this is Serge’s card sort, which gives students a chance to see both correct and incorrect uses of function notation.

Check out all of our activity additions here.

The Desmos Teaching Faculty has been thinking hard about modeling.

For too long, many students’ experience with mathematical modeling has been limited to problems that begin “The relationship between this and that can be modeled by the equation….”

Our goals are more ambitious. We want our tools to help students model even when they’re not using our tools.

Our work is partially informed by these excerpts from the Standards for Mathematical Practice:

Mathematically proficient students are comfortable making assumptions and approximations to simplify a complicated situation, realizing that these may need revision later.

They routinely interpret their mathematical results in the context of the situation and reflect on whether the results make sense, possibly improving the model if it has not served its purpose.

A new version of LEGO Prices represents our current best efforts here.

Students make a prediction about the price of a large LEGO set.

They sketch a relationship between price and number of pieces. The teacher can lead a discussion about those sketches—selecting and displaying ones that represent differing assumptions.

Then we impose a proportional relationship.

Students are free to argue back, and we hope that they will! In other modeling activities, we’ll ask them to select a model type or to build one from scratch. But at this early stage of modeling, we’re content to impose a structure in order to let students explore within these constraints.

We ask students to interpret the models they build, and to critique the constraints.

Finally, we build a little suspense by revealing the answer via video.

We’ll have a bunch of new and madeover modeling tasks in the near future. In the meantime, let us know how modeling is going in your classroom, and how we can strengthen our approach.

We just added support for multiple choice questions and video exhibits in our Activity Builder. These are the first features lots of companies add to their online activity platforms so we wanted to explain why we waited so long.

First, we wanted to get them right, to build them in alignment with our pedagogical principles. Multiple choice questions can offer meaningful assessment, but it’s also easy to answer them correctly without knowing much of anything and it’s easy to answer them incorrectly while knowing quite a lot.

So we took our time to integrate multiple choice with explanation. Students will select an option and they’ll also have to explain that selection. Students will also see explanations from three other students, consistent with our interest in connecting students and their thinking together.

These are our default options, too. We needed time to communicate our pedagogical preferences through design and code.

Second, we wanted to cultivate a teacher base and a house style. What interests Desmos more than many of our colleagues in this space is informal mathematical thinking. It’s harder for machines to automatically grade that kind of thinking but we know it’s the kind of thinking that often interests students in mathematics and it makes formal mathematics easier to learn. So we built a text box and we built sketch – two useful conduits for informal mathematical thinking – before we built multiple choice and video.

We also speculated that our earliest teacher base would come to define our company and challenge our ideas about mathematics education in essential ways. If multiple choice and video were the first features we built, we anticipated registering many teachers who were interested primarily in uploading video lectures and creating quizzes. We think Desmos is useful for those purposes and we welcome those teachers. But more than we need teachers who will challenge us to be an adequate Khan Academy, we need teachers who will challenge us to be the best Desmos.

We couldn’t be happier with the results of those efforts. Feature requests are a joy to receive more often than not because our teachers understand our project.

We’ve set a bar for ourselves. Our teachers push it higher and help us clear it.

Stay tuned.