Des-blog

The Friday Fave enjoyed a splendid Zero Derivative of Day Length Day this week, and hopes that you did too. In the Fave’s part of the world, the days are now getting shorter. Maybe in yours they’re getting longer. Either way, we all have Zero Derivative Day in common.

That got the Friday Fave thinking about daylight relationships more broadly, which leads the Fave to remind you of the activity Burning Daylight—this week’s Friday Fave.

If you are a math teacher with any affinity for applications or modeling at all (and that should be pretty close to everybody reading this right now), you probably see many beautiful things to notice in the following image, which compares Miami, Florida and Fairbanks, Alaska. Which is which?

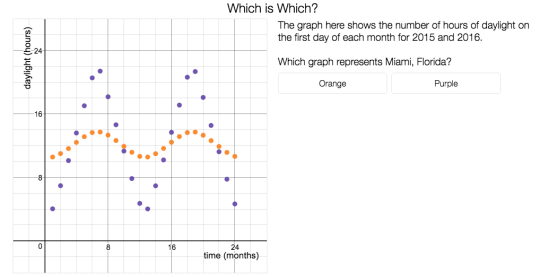
In Burning Daylight, this task launches students into the real question, which is “Which city gets more total daylight?” Calculus is a useful tool for answering that question, but not an essential one.

Students model with trig functions; they develop their own ways of answering that big question, and then we ask a terrific question to get them thinking more deeply about the connections between the math and the reality.

Barrow, Alaska is the northernmost city in the United States. What does the graph of daylight hours look like for Barrow?

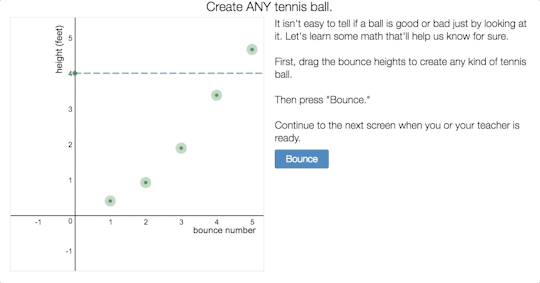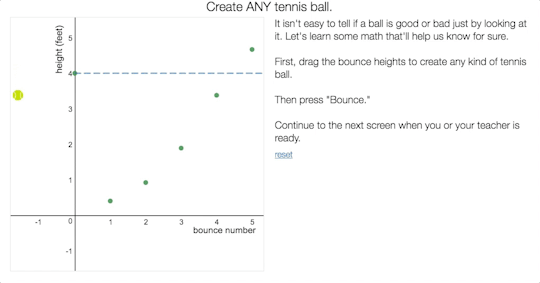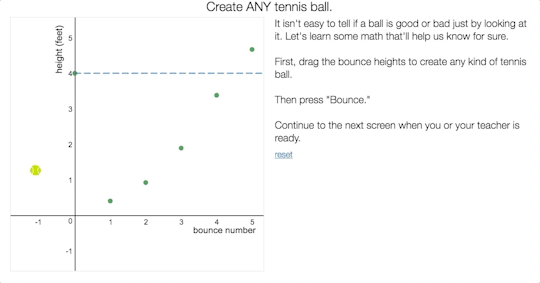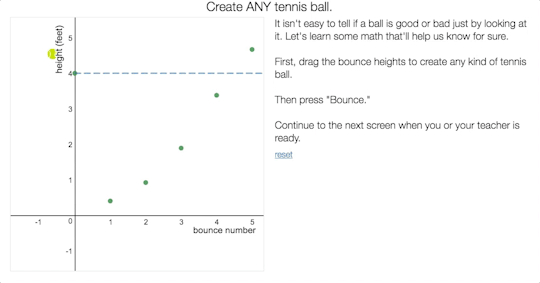
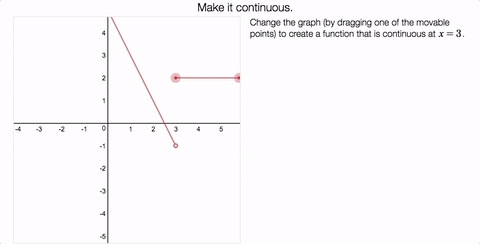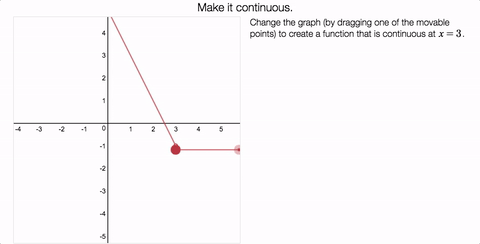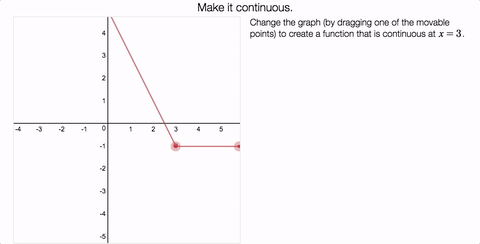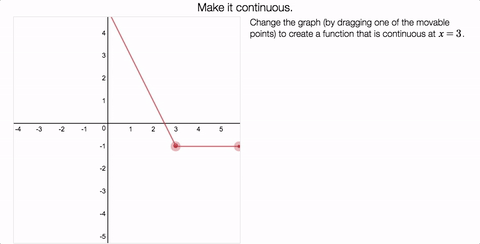
While you’re thinking about trig functions, here are a few other opportunities to play with sines, cosines, and all the rest:

Marbleslides: Periodics

Polygraph: Sinusoids

Trigonometric Graphing

This past week we asked the Desmos fellows to share a favorite Desmos activity and to tell why it worked for their class and how it helped students learn mathematics. The group generated a great list of activities and ideas, three of which we’ll highlight below.

Exploration

Desmos can help students explore concepts visually in a low risk environment. They can test out theories and adjust their current model of thinking accordingly. In Loco for Loci! we start by asking students to drag the green point in a graph so that it is four units from the blue point. Then we ask student to predict what it would look like if all of their classmates dragged the green point four units from the blue point. The teacher reveals the relationship by sharing the class overlay of all points.

Dylan Kane shares that “Students got to play with some points and make predictions, and I don’t know that a ton of learning happened in that first stage, but they had the chance to get some practice thinking about what a locus is (even if they didn’t have that language) as well as make predictions (most of which lacked precision). Then, at the end, we looked back at students’ work as a class and had a chance to formalize a definition of locus, as well as understand why different prompts resulted in different mathematical objects.”

Class Discussion

Kathy Henderson shares that “One of the most valuable outcomes of a good Desmos activity is when the activity allows students to recognize misconceptions of a topic and then allows class discussion of those misunderstandings.” Match My Parabola supports discussion by allowing students to explore the various forms of a parabola followed by an opportunity to reflect on what they’ve learned using various strategies to justify their thinking.

Connecting Representations and Visual Feedback

Neel Chugh shared that Game, Set, Flat “allows students to see the connections between geometric sequences and exponential functions really well. It also gives the the opportunity to conjecture and test out different ideas supported by great animation.”

How has Desmos helped your students learn mathematics? Let us know on Twitter @desmos.

If you’ve ever found yourself using your fingertips to trace the path of a point along a curve to help yourself or someone else think about the meaning of right and left-handed limits, then this week’s Friday Fave—Limits and Continuity—may just be your ticket.

Take all those continuity diagrams from your Calculus textbook and make them interactive. This was Bryn Humberstone’s mission in developing the activity originally.

All we did was apply a tiny bit of Desmos Activity Polish ™ and make it available to you.

NB: While students can interact with these diagrams equally well on laptops and on touchscreens, we cannot be held accountable for fingerprints they may leave on your devices.

While you’re in a Calculus sort of a way, here are three more faves:

The Intermediate Value Theorem

Average Value of a Function

Sketchy Derivatives